Theories based only on real numbers fail to explain the results of two new experiments
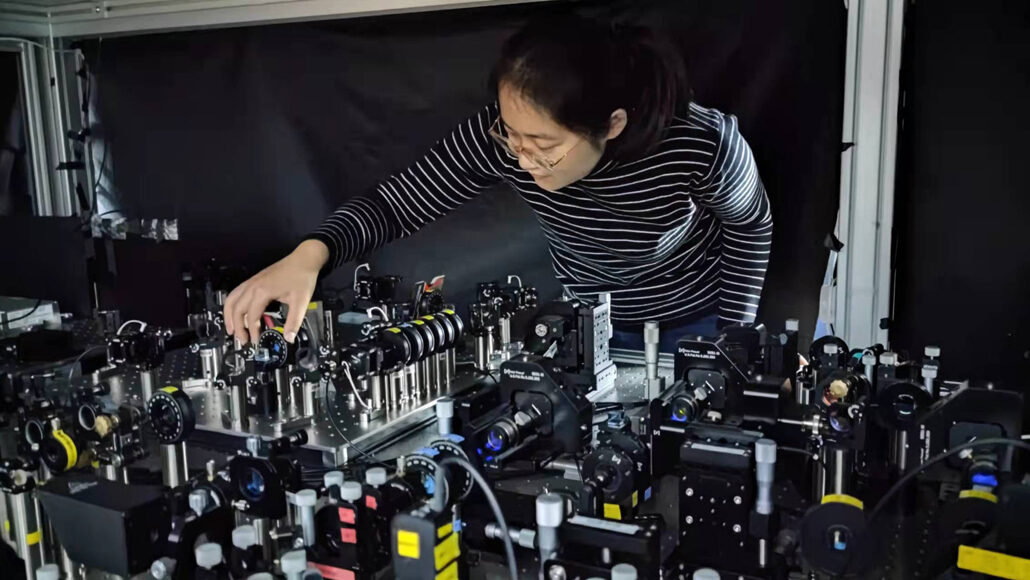
To explain the real world, imaginary numbers are necessary, according to a quantum experiment (shown) performed by a team of physicists including Yali Mao (pictured) of the Southern University of Science and Technology in Shenzhen, China.
JINGYUN FAN
By Emily Conover
Imaginary numbers might seem like unicorns and goblins — interesting but irrelevant to reality.
But for describing matter at its roots, imaginary numbers turn out to be essential. They seem to be woven into the fabric of quantum mechanics, the math describing the realm of molecules, atoms and subatomic particles. A theory obeying the rules of quantum physics needs imaginary numbers to describe the real world, two new experiments suggest.
Imaginary numbers result from taking the square root of a negative number. They often pop up in equations as a mathematical tool to make calculations easier. But everything we can actually measure about the world is described by real numbers, the normal, nonimaginary figures we’re used to (SN: 5/8/18). That’s true in quantum physics too. Although imaginary numbers appear in the inner workings of the theory, all possible measurements generate real numbers.
Quantum theory’s prominent use of complex numbers — sums of imaginary and real numbers — was disconcerting to its founders, including physicist Erwin Schrödinger. “From the early days of quantum theory, complex numbers were treated more as a mathematical convenience than a fundamental building block,” says physicist Jingyun Fan of the Southern University of Science and Technology in Shenzhen, China.
Some physicists have attempted to build quantum theory using real numbers only, avoiding the imaginary realm with versions called “real quantum mechanics.” But without an experimental test of such theories, the question remained whether imaginary numbers were truly necessary in quantum physics, or just a useful computational tool.
A type of experiment known as a Bell test resolved a different quantum quandary, proving that quantum mechanics really requires strange quantum linkages between particles called entanglement (SN: 8/28/15). “We started thinking about whether an experiment of this sort could also refute real quantum mechanics,” says theoretical physicist Miguel Navascués of the Institute for Quantum Optics and Quantum Information Vienna. He and colleagues laid out a plan for an experiment in a paper posted online at arXiv.org in January 2021 and published December 15 in Nature.
In this plan, researchers would send pairs of entangled particles from two different sources to three different people, named according to conventional physics lingo as Alice, Bob and Charlie. Alice receives one particle, and can measure it using various settings that she chooses. Charlie does the same. Bob receives two particles and performs a special type of measurement to entangle the particles that Alice and Charlie receive. A real quantum theory, with no imaginary numbers, would predict different results than standard quantum physics, allowing the experiment to distinguish which one is correct.
Fan and colleagues performed such an experiment using photons, or particles of light, they report in a paper to be published in Physical Review Letters. By studying how Alice, Charlie and Bob’s results compare across many measurements, Fan, Navascués and colleagues show that the data could be described only by a quantum theory with complex numbers.
Another team of physicists conducted an experiment based on the same concept using a quantum computer made with superconductors, materials which conduct electricity without resistance. Those researchers, too, found that quantum physics requires complex numbers, they report in another paper to be published in Physical Review Letters. “We are curious about why complex numbers are necessary and play a fundamental role in quantum mechanics,” says quantum physicist Chao-Yang Lu of the University of Science and Technology of China in Hefei, a coauthor of the study.
But the results don’t rule out all theories that eschew imaginary numbers, notes theoretical physicist Jerry Finkelstein of Lawrence Berkeley National Laboratory in California, who was not involved with the new studies. The study eliminated certain theories based on real numbers, namely those that still follow the conventions of quantum mechanics. It’s still possible to explain the results without imaginary numbers by using a theory that breaks standard quantum rules. But those theories run into other conceptual issues, making them “ugly,” he says. But “if you’re willing to put up with the ugliness, then you can have a real quantum theory.”
Despite the caveat, other physicists agree that the quandaries raised by the new findings are compelling. “I find it intriguing when you ask questions about why is quantum mechanics the way it is,” says physicist Krister Shalm of the National Institute of Standards and Technology in Boulder, Colo. Asking whether quantum theory could be simpler or if it contains anything unnecessary, “these are very interesting and thought-provoking questions.”
Questions or comments on this article? E-mail us at feedback@sciencenews.org
CITATIONS
M.-O. Renou et al. Quantum theory based on real numbers can be experimentally falsified. Nature. Published online December 15, 2021. doi: 10.1038/s41586-021-04160-4.
M.-C. Chen et al. Ruling out real-valued standard formalism of quantum theory. Physical Review Letters. In press, 2021.
Z.-D. Li et al. Testing real quantum theory in an optical quantum network. Physical Review Letters. In press, 2021.
About Emily Conover
Physics writer Emily Conover has a Ph.D. in physics from the University of Chicago. She is a two-time winner of the D.C. Science Writers’ Association Newsbrief award.
Quantum theory needs complex numbers

Physicists construct theories to describe nature. Let us explain it through an analogy with something that we can do in our everyday life, like going on a hike in the mountains. To avoid getting lost, we generally use a map. The map is a representation of the mountain, with its houses, rivers, paths, etc. By using it, it is rather easy to find our way to the top of the mountain. But the map is not the mountain. The map constitutes the theory we use to represent the mountain's reality.
Physical theories are expressed in terms of mathematical objects, such as equations, integrals or derivatives. During history, physics theories evolved, making use of more elaborate mathematical concepts to describe more complicated physics phenomena. Introduced in the early 20th century to represent the microscopic world, the advent of quantum theory was a game changer. Among the many drastic changes it brought, it was the first theory phrased in terms of complex numbers.
Invented by mathematicians centuries ago, complex numbers are made of a real and imaginary part. It was Descartes, the famous philosopher considered as the father of rational sciences, who coined the term "imaginary," to strongly contrast it with what he called "real" numbers. Despite their fundamental role in mathematics, complex numbers were not expected to have a similar role in physics because of this imaginary part. And in fact, before quantum theory, Newton's mechanics or Maxwell's electromagnetism used real numbers to describe, say, how objects move, as well as how electro-magnetic fields propagate. The theories sometimes employ complex numbers to simplify some calculations, but their axioms only make use of real numbers.
Schrödinger's bewilderment
Quantum theory radically challenged this state of affairs because its building postulates were phrased in terms of complex numbers. The new theory, even if very useful for predicting the results of experiments, and for instance perfectly explains the hydrogen atom energy levels, went against the intuition in favor of real numbers. Looking for a description of electrons, Schrödinger was the first to introduce complex numbers in quantum theory through his famous equation. However, he could not conceive that complex numbers could actually be necessary in physics at that fundamental level. It was as though he had found a map to represent the mountains but this map was actually made out of abstract and non-intuitive drawings. Such was his bewilderment that he wrote a letter to Lorentz on June 6, 1926, stating "What is unpleasant here, and indeed directly to be objected to, is the use of complex numbers. Ψ is surely fundamentally a real function." Several decades later, in 1960, Prof. E.C.G. Stueckelberg, from the University of Geneva, demonstrated that all predictions of quantum theory for single-particle experiments could equally be derived using only real numbers. Since then, the consensus was that complex numbers in quantum theory were only a convenient tool.
However, in a recent study published in Nature, ICFO researchers Marc-Olivier Renou and ICREA Prof. at ICFO Antonio Acín, in collaboration with Prof. Nicolas Gisin from the University of Geneva and the Schaffhausen Institute of Technology, Armin Tavakoli from the Vienna University of Technology, and David Trillo, Mirjam Weilenmann, and Thinh P. Le, led by Prof. Miguel Navascués, from the Institute of Quantum Optics and Quantum Information (IQOQI) of the Austrian Academy of Sciences in Vienna have proven that if the quantum postulates were phrased in terms of real numbers, instead of complex, then some predictions about quantum networks would necessarily differ. Indeed, the team of researchers came up with a concrete experimental proposal involving three parties connected by two sources of particles where the prediction by standard complex quantum theory cannot be expressed by its real counterpart.
Two sources and three nodes
To do this, they thought of a specific scenario that involves two independent sources (S and R), placed between three measurement nodes (A, B and C) in an elementary quantum network. The source S emits two particles, say photons, one to A, and the second to B. The two photons are prepared in an entangled state, say in polarization. That is, they have correlated polarization in a way which is allowed by (both complex and real) quantum theory but impossible classically. The source R does exactly the same, emits two other photons prepared in an entangled state and sends them to B and to C, respectively. The key point in this study was to find the appropriate way to measure these four photons in the nodes A, B, C in order to obtain predictions which cannot be explained when quantum theory is restricted to real numbers.
As ICFO researcher Marc-Olivier Renou comments "When we found this result, the challenge was to see if our thought experiment could be done with current technologies. After discussing with colleagues from Shenzhen-China, we found a way to adapt our protocol to make it feasible with their state-of-the-art devices. And, as expected, the experimental results match the predictions." This remarkable experiment, realized in collaboration with Zheng-Da Li, Ya-Li Mao, Hu Chen, Lixin Feng, Sheng-Jun Yang, Jingyun Fan from the Southern University of Science and Technology, and Zizhu Wang from the University of Electronic Science and Technology is published at the same time as the Nature paper in Physical Review Letters.
The results published in Nature can be seen as a generalization of Bell's theorem, which provides a quantum experiment which cannot be explained by any local physics formalism. Bell's experiment involves one quantum source S that emits two entangled photons, one to A, and the second to B, prepared in an entangled state. Here, in contrast, one needs two independent sources, the assumed independence is crucial and was carefully designed in the experiment.
The study also shows how outstanding predictions can be when combining the concept of a quantum network with Bell's ideas. For sure, the tools developed to obtain this first result are such that they will allow physicists to achieve a better understanding of quantum theory, and will one day trigger the realization and materialization of so far unfathomable applications for the quantum internet.Researchers investigate 'imaginary part' in quantum resource theory
More information: Miguel Navascués, Quantum theory based on real numbers can be experimentally falsified, Nature (2021). DOI: 10.1038/s41586-021-04160-4. www.nature.com/articles/s41586-021-04160-
Journal information: Nature Physical Review Letters
Provided by ICFO
No comments:
Post a Comment